DIFFERENTIAL SENSITIVITY/LIMEN IN

                   

1. ·      FREQUENCY
2. ·      INTENSITY
3. ·      TIME

INTRODUCTION

The ear’s extremely wide range of sensitivity is one of the most striking aspects of audition. The ear is sensitive to range of intensities from about 0 dB SPL (which is amplitude of vibration of about size of a hydrogen molecule) to roughly 140 dB (at which pain and damage to the auditory mechanism ensue).This dynamic range of the approximately 140 dB corresponds to pressure ratio of 107:1. In other words, the most intense sound pressure that is bearable is on the order of 10 million times as great as the softest one that is perceivable under optimum listening condition. In terms of frequency, humans can hear tones as low as 2 Hz (although roughly 20 Hz is required for a perception of “tonality”) and as high as about 20 KHz. The frequency and intensity sensitivities of ear can interact, affecting each other to a greater or lesser degree. In addition, when duration the duration of sound is less than about half of a second, it affects both frequency and intensity sensitivity. Longer duration may be thought of as being infinitely long as far as auditory sensitivity is concerned.

The ear is able to discriminate small differences in a wide range of stimuli, i.e. it has a remarkable sensitivity- the ability to detect very small differences between similar sounds. This ability applies to all three parameters: intensity, frequency, and time.

DIFFERENTIAL SENSITIVITY   

It is the smallest perceivable difference between two sounds. This quantity is called either the difference limen (DL) or the just noticeable difference (JND). The DL is the smallest perceivable difference in db between two intensities (∆I) or the smallest perceivable change in Hz between two frequencies (∆f).

We may think of JND in two ways.

·         The absolute difference between two sounds

·         The relative difference between two sounds

Absolute difference is how much sound intensity is necessary for a typical, normally hearing person to just detect the presence of a stimulus or it is the least sound pressure that leads to a sensation of hearing.

Relative difference is obtained by dividing the absolute DL by the value of starting level. Thus, if the starting level is 1000 units and the DL ∆I/I is 50/1000=0.05. This ratio, ∆I/I, is called the Weber fraction.

The frequency DL ∆f is an absolute difference in Hz, as opposed to the relative frequency DL obtained by dividing ∆f by the starting frequency f. Suppose it is necessary to change a 1000 Hz tone (f) by a 3.6 Hz ∆f in order for a particular subject to just detect the frequency difference. His absolute frequency DL is thus 3.6 Hz, whereas his relative DL is 0.0036.The situation is different, however, for the intensity DL, because we measure in dB.

 DIFFERENTIAL SENSITIVITY FOR INTENSITY

Successive and simultaneous comparison

There are two quite distinct tasks can be posed to the listener when investigating the discrimination of changes in intensity. The listener’s memory requirements are very different in these two tasks and the results obtained with these two tasks and the results obtained with these two tasks are quite different. In the classical approach to intensity discrimination, the sounds are presented successively to the listeners. In effect a pair of waveforms, f (t) and K.f (t) are presented. Where K is constant. The discrimination task amounts to determining the order in which the pairs are presented, loud soft or soft loud. Since the sounds are presented in succession, the listener must retain some memory of the first estimate so that it can be compared with later estimate.

A second way to study intensity discrimination is to require the listener to compare at least two sounds that are presented simultaneously. The memory processes required minimal. The listener was asked to compare at least two spectral regions of a single sound. Often, two sounds are presented in one of the spectral levels are the same, in other, they differ. Green, Kidd & Picardi (1983) have shown that interstimulus interval has very little effect on the result obtained in such experiments. Their results also showed that simultaneously intensity comparison were often more sensitive than successive comparisons.

FACTORS AFFECTING DLI

1. INTENSITY

  

WEBER’S LAW

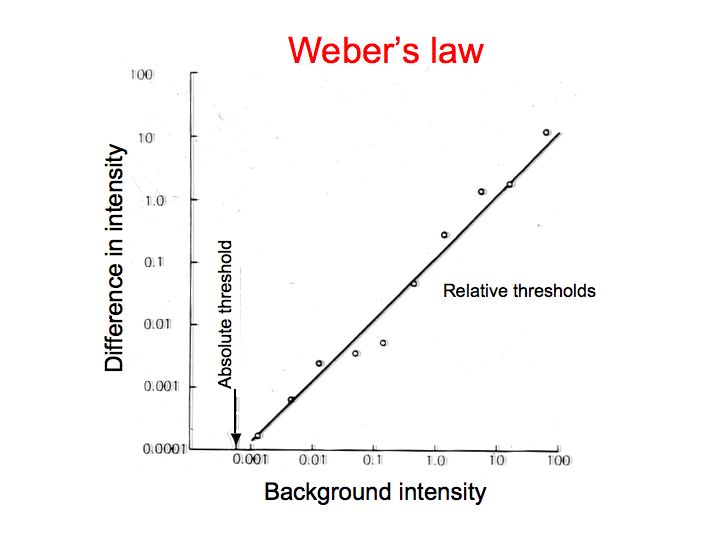
The important concept in psychophysics is Weber’s law (1934).It states that the value ∆I/I=K i.e. Weber fraction is a constant regardless of the stimulus.E.g.to lit a number of candles already present you have to add more to perceive a difference in the amount of light. If 10 candles are originally lit, then only one more lit will produce JND of light. Thus, absolute value of DL increased from 1 to 100 where as K remains constant at 0.1 (1/10, 10/100, 100/10000).

Fetcner (1960) criticized Weber’s law and reported that the constant fraction decreases as the base intensity increases, which is called near miss Weber’s law.

Reisz (1928) observed that ∆I/I decreases with increase in sensation level. He reported differential sensitivity for intensity for a wide range of frequency (35 Hz to 1000Hz) and sensation level (0 to 100 dB). He used amplitude modulation to determine DLI. The threshold determined by listeners adjusting the amplitude until they could just hear fluctuation in amplitude. The intensity difference between two tones was taken as measure of DLI. Results showed ∆I/I is frequency dependent as it becomes smaller from 35 Hz to 1 KHz. Above this frequency, Weber fraction remains constant above 20 dBHL.

FIG.1

 

PURE TONE INTENSITY DSCRIMINATION

Some experiments relating to the near miss Weber’s Law:

A number of researchers have shown the Weber’s law does not hold for DLI .Instead, it is found that if ∆I is plotted against I, a straight line is obtained with a slope of 0.9. Thus discrimination improves at high levels. This has been termed near miss to Weber’s law.

Adding high pass noise generally decreases or eliminates near miss and data are consistent with Weber’s law that is ∆I/I becomes independent of the standard (Viemeister 1972, Moore & Raab 1974, Florentine 1983).

Weber’s law is characterizes the behaviors of single channel and head near miss results from intensity dependent spread of excitation, primarily to frequency region higher than that of standard. If cochlear damage is moderate, the response to the standard of a channel tunes above the frequency of the standard may be normal, that is when the OHC damaged to low frequency falls of tuning curves essentially normal. Thus spread of excitation may be normal, resulting in normal intensity discrimination. If the damage is severe at frequency regions above the frequency of the standard, as in the cases of IHC damage, the response of the channels turned to these regions will be abnormal or non existent. Then ∆I/I will be impaired.

2. FREQUENCY

Walt Jessteadt, Craig C Wiet, David M Green (1976) studied the intensity discrimination as a function of frequency and sensation level. They used pulse sinusoids of various frequencies (200-8KHz) is sensation level of 5 to 80 dB. Intensity discrimination was measured at intensities of 5, 10, 20, 40, & 80 dBSL for frequencies 400, 600, 800, 1000, 2000 and 8000 Hz. Subjects tested at lowest frequency and moved towards the high frequency from high to low sensation level.

Results showed ∆I/I decrease linearly as a function of SL which is described as near miss to Weber’s law. But no effect of frequency and no interaction of sensation level with frequency. Results of this study differed from several previous studies. Previous studies Mc Gill et al (1968), Campbell et al (1967) found at low levels DLI is non linear. This study found clear derivation of DLI from linear function only for signals less than 5 dBSL.

Mary Florentine (1983) studied how intensity discrimination depends on the test frequency, the level and a subject’s high frequency hearing. She performed three experiments.

1. In the first experiment intensity discrimination of pulsed tone was measured as a function of level at 1 & 14 kHz.

2. In the second experiment intensity discrimination was measured for a 1 kHz tone at 90 dBSL as a function of the cut off frequency of a high pass masking noise.

3. In the third experiment, threshold from 6 to 20 kHz and intensity discrimination for a 1 kHz was measured.

To examine the frequency dependency of intensity DLs and established the DLs as a function levels, the measurement made at many levels from the threshold of audibility to the near threshold of discomfort. To show the effect of frequency, the DLs were measured at 1 and 14 KHz. At each SL several increments were chosen to yield 60 to 90% correct responses. The subject’s task was to press the button corresponding to the interval in which the more intense stimulus was presented.

RESULTS:

DL improved less with increasing level at 14 KHz than at 1 KHz.

DLs at 14 KHz are clearly larger than the DLs at 1 KHz except at the lowest sensation levels.

All subjects showed improved DLI with increasing level at 1 KHz but little improvement with increasing level at 14 KHz.

DISCUSSION: DLs at high levels are smaller for 1 KHz tone than for 14 KHz tone because the 1 KHz tone spreads its excitation over a wide rage especially towards the high frequency. .     

3. EFFECTS OF PHASE DIFFERENCE OF STIMULUS ON DLI

Green and Mason 1985 compared the detection of an increment in the central component of a multi tonal complex in two phase condition. In the first the phase of all components of the complex were fixed. When it is fixed the standard stimulus had same wave shape. In the second condition the phases of each components of the complex were randomly selected for each presentation and hence the wave form was unpredictable. Only the difference in the power spectrum distinguishes the standard from standard plus signal. The results showed that the threshold for these two signals with addition of another signal were same. Hence it is concluded that the phase of the signal is irrelevant in detection of DLI.

4. EFFECTS OF STIMULUS VARIABLE ON DLI

The figure shows that the continuous presentation method (solid circle) was slightly different from those obtained from gated presentation method. The continuous thresholds are larger at the lowest sensation level than the pulsed presentation. Thus, the stimulus variable known to affect the size of the DL (Green and Nachmlas 1979)

5. EFFECTS OF STIMULOUS DURATION ON DLI

Riezes (1928) first studied the effect of duration of the stimulus on difference limen. This study later followed by Garner and Miller 1944, Henning 1970 and Florentine 1966. These studies repots that the ability to hear small changes in intensity improves as the duration of the stimulation increases.

Florentine (1986) summarized the effect of stimulus duration on DLI suggesting that Dl decreases with shallow slope until duration of 1 second or more is reached, the DL is constant or increases very little with further changes in duration. The relation holds for frequencies 250, 1000, and 8000 Hz at 40, 65, and 85 dB SPL. Berliner, Durach and Braida (1977) also measured improvement in the difference limen as signal duration changes from 0.5 to 1.4 seconds. They suggest that the ear has a relatively long integration time for an intensity increment in sinusoidal signal.

6. MASKING NOISE

(Miller 1947; Viemster 1972; Moore and Raab1974), under most listening condition there is simple relation between the intensity of a sound and DLI. For tones in noise background, the Weber function is constant over a wide range of base line level (Weber law), where as tones presented in isolation, Weber fraction decreases with increasing level.

7. MEASUREMENT TECHNIQUE

i. Modulation detection: Listeners are required to detect the presence of slow amplitude modulation (AM), threshold being taken as the smallest detectable depth of AM.

ii. Increment detection: Listeners are required to detect a change in the intensity of standard stimulus. Standard can be presented either continuously or gated with the increment.

Intensity discrimination at 1 kHz in the presence f high pass noise:

(Florentine, 1983) To test this DLI at 1 kHz tone at 90 dB SPL was measured in quiet and in the presence of high frequency noise. These results suggest that changing the cut off frequency of the masking noise from 6 to 19 kHz decrease the DLI at 1 KHz by a factor of 1.5 to 2.

MODELS

1. SINGLE BAND EXCITATION MODEL

Zwicker (1967): This model is based on excitation patterns that are derived from masking patterns. It assumes that the acoustic energy is transformed into pattern of excitation along the basilar membrane. Intensity discrimination is performed by discriminating changes in the excitation level that occurs as a consequence of a change on stimulus level.

He worked with AM tones and explained his results in terms of a non linear growth in the excitation pattern encoded by the stimulus. He assumed that performance was determined by the critical band in which the excitation grows most rapidly with stimulus level. He suggested that a change could be detected whenever the excitation pattern changed some where by 1 dB or more. At high levels the high frequency side will be produced by relatively smaller stimulus increments at high levels. In support of this idea, Zwicker reported that the addition of high pass noise, which masks high frequency side of the excitation pattern, causes the end for the amplitude modulation at high intensities to approach that found low levels

2. MULTIBAND VISION OF THE EXCITATION PATTERN:

It is derived by Florentine and Blass (1981) from the Zwicker model. This assumes that acoustic energy is transformed in to a pattern of excitation along the basilar membrane.

Intensity discrimination is performed by discriminating changes in the excitation level that occur as a consequence of a change in stimulus level. According to this model discrimination is performed in each 24 critical bands and then an optimum decision is formed on the basis of information in all critical bands.

FIG4

At higher SLs excitation will be above threshold over an increasingly larger frequency range and more critical bands become usable for intensity discrimination. Because of this there is better discrimination at higher intensity levels. At high levels excitation pattern is very broad and the excitation at frequencies for above the test frequency is important for intensity discrimination. Thus for example, DLs for intense 1 kHz tone depend on the absolute threshold at frequencies for above the test frequency.

This model also predicts that DLs at high levels also depend on frequency. For every high frequencies of sufficient intensity the excitation pattern will encompass all available critical bands towards the high frequency and the number of active critical bands will increase little with level. Hence, information from fewer critical bands is combined for high frequencies than for mid frequencies. Hence, poor DLI is predicted for frequencies exceeding 8 kHz than for middle frequencies.

This model is based on five assumptions;

1. The excitation pattern derived from Zwicker’s masking pattern model.

2. Excitation level discrimination in each of 24 critical bands is independent of the     excitation level discrimination in other critical bands.

3. Sensitivity of excitation level difference within a critical band is independent of excitation level, i.e. Weber law holds within each critical band.

4. Sensitivity is same or constant in all critical bands.

5. Information in critical bands is used according to a decision rule. The single and multi band version differ only by decision rule, which determine how the available information is used.

3. NEURAL COUNTING MODEL

Mc Gill and Goldberg (1968) presented an account of intensity discrimination upon neural counting mechanism. They suggested that stimulus energies are mapped on the neural impulse whose number fluctuates from trial to trial even for non varying stimuli. If the mean number of neural counts were proportional to stimulus energy this would lead to a number of neural counts generated in response to it non-linearly. This relation can be characterized as a power function of the form N=K (I/Io).

Luce and Green (1974) assumed that the system counts neural impulses or monitors their inter arrival times (IAT) over a number of channels. The IATs are assumed to be independent of one another with a distribution that is geometric with an additive error. This model of neural activity differs from the model proposed by Mc Gill and Goldberg, in that it assumed multi-model distribution of IAT for low frequency sinusoidal stimuli. In this study there are two sets of predictions.

1. Assuming intensity discrimination is based on the duration of IATs (timing model)

2. It is based on the total number of spikes in fixed time (counting model)

The authors have demonstrated that the particular timing model investigated predicts that ∆I/I increase with intensity, which is an obvious disagreement with the data.

The key assumptions are that the number of counts is related to intensity by power law and that the number of intensity and frequency.

Viemeister (1972) has proposed a model to account for the deviation from Weber’s law in terms of detection of distortion products at harmonics of the signal frequency. According to this model the near miss reflects the observer’s use of information from aural harmonics. At high levels, a given change in stimulus levels (in dB) produces a somewhat larger change in level (in dB) of one or more of the aural harmonics. He investigated the intensity discrimination of the pulsed sinusoids (f=950 Hz) in the presence of various types of filtered noise level was 6dB higher than the tone. He found little effect with low pass noise whose cut off frequency was 800 Hz, but a band pass noise with cut off at 1900 Hz both degraded performance at high intensities with later stimulus Weber’s law was obtained. Moore and Raab (1973) evaluated the Viemeister’s hypothesis using low levels of noise tonal maskers. They assessed DLI at low and high sensation levels, i.e. 36 dB and 75 dB. The DLI of 1000 Hz pure tone was studied under different conditions.                  

 Results: Experiment using tonal maskers: 1 KHz tone burst was presented together with phase lock 2000 Hz tone of fixed intensity. Assuming that 2000 Hz stimulus should affect the second aural harmonics they hypothesized that when 2 KHz tone level is lower than the second harmonics there should be no or little effect on DLI. When 2 KHz level is higher than the aural harmonic, the aural harmonic  will no longer provide useful cues, and DLI high levels should be impaired . When these hypotheses were tested 2 KHz tone did not show any effect on DLI. Thus constant phase does not seen to be important in this situation, i.e. it is difficult to impair intensity discrimination at high levels with stimuli which are specially designed to eliminate information associated with aural harmonics.

Experiments using Noise makers: Viemeister (1972) demonstrated that low pass noise had little effect on intensity DLs, that high pass noise produced a deterioration n performance with high intensity levels.

Moore and Raab studied the effect of high pass noise and wide band noise on DLI. They observed that smaller DLs at high levels than at low level even though the noise levels are unchanged by the addition of high pass noise. The noise also reduced decrement in performance at high levels, but the decrement is not as large as for high pass noise. Thus information at frequencies above the frequency of the tone does seem to be important for important discrimination at high levels. However, the failure to eliminate the near miss compliably with the high pass noise could indicate that information on the low frequency side was also used. This was investigation using band pass noise. The result shows that discrimination performance at high levels was worse than was found for the high pass noise and the subject’s exhibited good approximation to Weber’s law. Thus it is concluded that both high and low frequency information taken together, this experiment show that subject’s use of information on the low frequency side will be more easily reduced when information on the high frequency side is removed.

Criticism:

According to this study results, explanation of the near miss in terms of aural harmonics is not appropriate. Zwicker’s model fails to explain low frequency side of the test tone. It explains only the importance of high frequency side.

INTENSITY DISCRIMINATION IN NORMAL HEARING AND HEARING IMPAIRED LISTENERS

Schroder, Viemeister and Melson (1994): According to these authors depending on the severity and configuration of hearing loss persons with cochlear loss show normal or elevated Weber function at given SPL, which is below sensational level because of relatively high SPL, the cochlear hearing impaired ear may utilize the larger spread of excitation. The recent studies shows that such cases show near miss (Schluch and Wier 1987, Turmer et al 1989, Florentine 1993). These studies indicate in cochlear hearing impaired the Weber fractions at high SPLs are approximately equal to or larger than those from normal. But at comparable SLs it is smaller than normal.

Comprehensive data, using many test levels and frequencies are obtained from listeners with flat and higher frequency losses and from normal hearing listeners. To assess the possible role excitation, the effects of high pass noise in both cochlear and normal listeners are examined.

FLAT HEARING LOSS

Figure shows results from the two listeners with flat hearing loss. These listeners had absolute threshold around 40 dB SPL. Weber fractions obtained in quiet from these two hearing impaired listeners fell near the mean normal curve in quiet. At 0.3 and 0.5 KHz for listener F1 the Weber fractions obtained in quiet from these two hearing impaired listeners fell near the mean normal curve in quiet. At around 0.3 and 0.5 KHz for listeners F2 the Weber fractions were slightly below the mean curve but still within the normal range.

Thus, when compared to equal SPLs the Weber fractions, for these hearing impaired listeners with flat hearing losses are approximately the same as those from normal hearing listeners. This data suggest that spread of excitation may be involved in intensity discrimination by these pair of listeners. The frequencies below 2 KHz the data indicate normal Weber fractions at high levels and elevation in the Weber fraction produced by the addition of high pass noise. However it is not clear whether listeners show the near miss.

HIGH FREQUENCY HEARING LOSS

Figures show from the 3 listeners with high frequency hearing loss. Results at the lower frequencies within the regions of normal hearing for listeners H1 and H2 were consistent with expectations based on the normal hearing data. The near miss was evident in quiet for listeners H1 at 0.3, 0.5 and 1 KHz and for H2 at 0.3, 0.5 KHz. Although listener H3 showed the near miss at 0.3 and 0.5 KHz where normal hearing existed, the case did have larger Weber fractions than the normal hearing listeners. Except for H1 the data for test frequencies immediately adjacent to and within the region of loss showed elevated Weber fractions at high level and for H2 no effect of high pass noise. This indicates that spread of excitation was not involved in intensity discrimination at these frequencies. The listener H1 shows normal Weber fraction in quiet and elevated Weber fractions in high pass noise at 2 and 3 KHz suggesting that despite a steep high frequency loss there was usable excitation above the test frequency.

DISCUSSION:

When compared the literature Weber fractions obtained for normal in quiet were in agreement with those reported by the studies. The near miss to Weber’s law observed at all test frequencies. For the range of frequencies examined in this study (0.3 to 3 KHz) there was also a trend for the Weber fraction at high levels to be slightly smaller at higher frequencies. The addition of high pass noise reduces the DLI. The results were consistent with the notion that the higher frequencies showed normal Weber fractions and tented to show the near miss. Those who had elevated threshold at high frequency showed limited use of excitation and elevated Weber fraction at a given SPL and tended to show Weber’s law. The data obtained with high pass noise shows the listener with cochlear impairment shows spread of excitation and the audiological information was effectively restricted to a very limited frequency region. This indicates that normal Weber fraction in cochlear haring impairment high level is due to usual sensitivity.

COMPARISON AT EQUAL SL:

Many earlier studies (Luscher and Zwislocki 1949; Danes and Nauton 1950) speculated that in cases of cochlear hearing loss due to abnormal growth of loudness the intensity discrimination should be better than normal. Clinical test for abnormal loudness growth were developed based upon this premise (E.g. SISI, Jerger et al 1959). In this study it is shown that in regions of cochlear hearing loss intensity discrimination at a given SPL can be normal or near normal. This implies that Weber fraction for impaired ear at a given SL can be normal or near normal, i.e. the DLI can be around 8 dB smaller than those of normals at equal SLs. It supports that the hearing impaired use large of spread if excitation at high SPLs than normal, a decreased or improved DLI is not due to the loudness recruitment. The diagnostic test based on this is not valid, but such tests are clinically useful still. The present study also shows that Weber fraction elevated in regions in regions of hearing loss. This can be also explained based upon spread of excitation because in case of increased Weber fraction recruitment is present but DLI is poor. So, elevated DLI do not imply an absence of recruitment.

INTENSITY DISCRIMINATION AT LOW LEVELS

It is well known that slope of rate versus level function of normal auditory nerve fibers increases as the frequency of the stimulating tone decrease below the characteristic frequency (Sachs and Abbas 1974, Harrison 1984). It is also shown that frequency dependency is largely eliminated in cases of cochlear pathology and specifically that the rate versus level function for HF tones is considerably steeper than normal (Harrison 1989).

The steeper rate Vs level functions seen in cochlear pathology also suggest the possibility that Weber fractions near detection threshold might be unusually small i.e. a smaller intensity change is necessary to produce a given change in rate. But the relationship between intensity discrimination and loudness is highly uncertain.

The figure compares fractions obtaining high pass noise for listeners with flat and high frequency hearing loss (open squares) with the mean Weber fractions for normal hearing listeners in quiet (solid lines). Weber fractions obtained up to 25 dB SL are shown. This data indicates smaller Weber fractions for cochlear impaired listeners. The average difference for levels below 20 dB SL is 2.3 dB. For the high frequency loss listeners at frequencies for which the loss is substantial, the Weber fractions are smaller than normal.

The results of this comparison are consistent with expectations based on steeper rate versus level functions in regions of cochlear loss. There are several aspects which prevent strong conclusion from this comparison because we cannot be sure that high pass noise restricts information to a region comparable to that for normal at low levels. Thus the smaller Weber fractions may reflect incomplete elimination of the near miss. Physiological data also shows Weber fractions are much smaller than those from normal. For levels below 20 dB SL the corresponding level differences are 2.3 dB and 1.5 dB respectively. That is 2.3 dB increase for the normal should produce the same change in rate that a 1.5 dB change produces in the cochlear impaired listeners. For this to be true the ratio of the slope of the rates Vs level function would be 2.3/1.5=1.5 a value considerably less than the ratio of approximately 5 indicated by the auditory nerve data (Harrison 1981). Finally the fact that the rate Vs level functions are steeper regions of pathology does not in itself imply that the Weber fractions should be normal. Unfortunately, the available data do not permit assessment of this possibility.

Summary:

Cochlear hearing loss – Weber fraction may be normal, if it is normal, with high pass noise it gets elevated, suggesting spread of excitation. When the Weber fraction is elevated, no further elevation in threshold with the high pass noise suggesting inability to use spread of excitation.

1.      In the regions of loss, the Weber fraction may be with normal range, but the near miss may no be evident in the Weber fraction with the high pass noise, suggest an inability to use spread of excitation.

2.      At a given SL, the Weber fraction for the frequencies in regions of cochlear loss may be considerably better than normal. In general this simply reflects the use of spread of excitation.

3.      At low SLs in high pass noise where excitation spread is limited the Weber fraction for frequencies in regions of cochlear loss are slightly better than normal.

Mary Florentine et al (1993) studied systematic measurements of intensity resolutions for pulsed tones as a function of levels in listeners with SN hearing loss. Particular attention was given to the test frequency relative to the configuration of the hearing loss, one listener with vestibular schwanoma and 6 listeners with normal hearing. Measurements were also made in normal ears presented with the masking noise separately shaped to produce audiograms similar to those of cochlear impaired listeners. For unilateral impairments, tests were made at same frequencies in the normal and impaired ears. For bilateral sloping impairments tests were made at different frequencies in the same ear. The normal listeners showed results similar to other data in the literature. The listeners with vestibular schwanoma showed greatly decreased intensity resolution except at few levels.

For listeners with recruiting SN impairments the results are discussed according to the configuration of the impairment and are compared across at equal SPL, equal SL and equal loudness level .Listeners with increasing hearing losses at frequencies above the test frequency generally showed impaired resolution, especially at high levels and less deviation from the Weber’s law than the normal listeners. Listeners with decreasing hearing loss as frequencies above the test frequency showed nearly normal intensity resolution functions. Where as these trends are generally present, there are large differences among individuals. Results obtained from normal listeners who were tested in the presence of masking noise indicate that elevated thresholds and reduced dynamic range account for some but not all of the effects SN impairment on intensity resolution.

INTENSITY DISCRIMINATION WITH COCHLEAR IMPLANTS

Intensity discrimination data from human implant patients have been difficult to interpret owing to a number of factors including the scarcity of data, the lack of independent indices of how well the subjects can perform an intensity discrimination task or how much very might be expected to improve with practice and the lack of adequate stimulus specification.

One of the more extensive tests of intensity discrimination in patients with cochlear implant was conducted by Bilger 1977 who tested 12 patients using acoustic stimuli delivered to the subject’s portable stimulator. Intensity discrimination was tested for 3 narrow band noise stimuli at various SL s ranging from 7 to 25 dB SL. DL ranged from 0.66 dB to >12 dB, but generally were 1.6 dB or smaller. In most of the cases they were smaller for low frequency noise (centered at 250 or 500 Hz) than for higher frequency noise (centered at 2 KHz). 3 subjects showed smaller difference limen for lower than for higher intensities of stimulation. 5 subjects showed neither frequency nor level dependence.

Intensity discrimination testing with known charges delivered to the electrodes has been done on 3 patients with modiolar implants (Simmons 1996, Mathews 1978, Walker 1978). A variety of intensities and repetition rate of pulsatile stimulation were used to test these patients. Intensity difference limens ranged from 0.16 to 8 dB averaging about 1.5 to 3 dB. The data are variable and there is no clear and consistent relation between the difference limen and with the intensity or repetition rate.

Much lower values were obtained by Hochmair 1981 who measured intensity difference limens in 5 patients with scala tympani implants and observed values ranging from 0.07 to 0.34 dB averaging about 0.2 dB.

CLINICAL APPLICATION OF DIFFERENTIAL SENSITIVITY FOR INTENSITY

The difference limen tests of recruitment are based on the measurement of the rate of change of loudness as the intensity increases. The rate of change with increase in intensity is greater in resulting than non-recruiting ears. The change in intensity in dB which results in just-barely-noticeable loudness change is termed the intensity difference limen for loudness.

     

 Methods of measuring DLI:

      Luscher-Zwislocki Method (1948)

They used pure tones, presented at 40 dB SL and patients were asked to indicate when amplitude modulation of steady state signal resulted in pulsating sound. Those patients who could detect small intensity change were assumed to have cochlear lesions. That is persons having a critical percentage modulation of less than 80% were considered to have recruitment.

   Limitations:

1.      Persons with mild or non- steepy sloping audiometric configuration obtained critical modulation percentage greater than 8% and some normal hearing persons obtained  less than 8%

2.      Individual variability in judgment affected the reliability of the test.

Denes-Naunton Method ( 1950)

They developed a recruitment test based on the intensity DL for loudness. In the test, two tones of the same frequency are presented to an ear and the intensity of one of the tones is varied until a perception of a just-noticeable change in loudness results. Test was conducted at 4 and 44 dB SL.

The determination of recruitment was based on a relative than absolute measure of the intensity DL for loudness .If the intensity DL increased or remained the same as the SL increased, recruitment was assumed to be present. If the intensity DL decreased as the SL increased as the SL increased, recruitment was assumed to be absent. In case of cochlear hearing loss not much difference at 44 dB SL and 4 dB SL, where as in normal at 44 dB SL -1 dB increment enough, at 3-4 dB SL increment is needed to detect the change. Jerger (1952) modified the Luscher-Zwislock test. He used presentation level at 15 dB SL rather than 40 dB SL, since the literature shows that the intensity DL was most affected between  10 and 30 dB SL. He found that this showed good differentiation between recruiting and non recruiting ears.

He found that patient with reduced intensity DLs had non conductive hearing impairments; enlarged intensity DLs had functional hearing loss, normal intensity DLs had conductive or presbycusis.

Luscher (1951) modified the intensity DL test to employ a presentation level of 80 dB HL. He employed in person with hearing loss magnitude exceeding 60 dB HL, the rationale underlying this modification two folds.Recruitment for hearing loss was present at this level is small in normal hearing person, indicating that the task of masking loudness judgments was simplified. Complete recruitment was assumed to be present if hearing impaired person obtains an intensity DL of 4-6% at this presentation level

DIFFERENTIAL SENSITIVITY FOR FREQUENCY

 Frequency discrimination in the hearing system is the ability to distinguish two non simultaneous sounds which differ on frequency but otherwise equal. It is important for many purposes to determine accurately the minimum change in the frequency of a tone which can be detected by the ear. It can be expressed in absolute or relative terms.

Absolute Method:

It is measured as the difference between the two frequencies in which the difference is perceived (unit= Hz)

Relative Method:

This is a method where the relative difference is obtained by dividing the absolute DL by the value of frequency at which it is being measured. E.g. suppose it is necessary to change a 1000 Hz tone (f) by a 2.6 Hz (f) in order to just detect the frequency difference then the absolute frequency DL is 2.6 Hz and the relative difference ∆f/f=2.6/1000=0.0026 Hz.

There are 3 ways of measuring frequency discrimination

1.      Difference Limen for Frequency (DLF)

It involves the discrimination of successive steady tones with slightly different frequency. This requires both use of short term memory for pitch and an ability to determine the direction of frequency change (Harris 1952; Jestead et al 1975).

2.      Frequency Modulation Difference Limen (FMDL)

It uses tones that are frequency modulated at low rate (typically 2 to 4 Hz). Here the subject can detect any change regardless of direction within the modulated stimulus. When this method is used, the frequency changes between f-∆f and f+∆f. Thus, the value for the total variation in frequency is 2f.

3.      Difference Limen for Change (DLC)

Here the task is to identify the interval in which the tones are different in frequency i.e. the subject has to detect any change regardless of the direction of the change in frequency.

FACTORS AFFECTING DLF:

Frequency discrimination as a function of frequency and test stimuli:

The early work of differential frequency sensitivity was plugged by Shower and Biddulph (1931). They used frequency modulated tones as the stimuli. A rest tone was varied continuously in frequency at a rate of 2/sec. The subject’s task was to detect the presence of a modulated as opposed to a steady tone. The DL was taken as the smallest difference in frequency that produced a perceptible modulation of original tone. The study included wide range of frequencies from 62 Hz to 11700 Hz and 5 to 80 dBSL. Result showed FMDL’s tend to vary less with frequency. However, they observed DL becomes larger as frequency increases and DL becomes smaller as SL increases.

Wier et al (1961), did a study using pulsed tone from 200 Hz to 8 KHz at 5 to 80 dBSL. The results showed DL becomes larger as frequency increases and DL becomes smaller as SL increases. The best value of DL occur at low frequency presented at about 40 dBSL or more.      

Moore and Sek (1994) measured frequency discrimination for a wide range of center frequency (0.25 to 8 KHz) using DLF, DLC and FMDL’s. The result showed for frequencies up to 2 KHz, DLF’s and DLC’s were small (less than 0.6% of center frequency) and were similar to one another. For frequencies of 4 KHz and above both DLF and DLC increased markedly but the increment was greater for DLF’s which indicates the worsening of performance at high frequencies is greater when subjects are required to indicate the direction of the frequency change than when they just have to detect any change. FMDL’s when expressed relative to the carrier frequency, varied much less with frequency than DLF’s or DLC’s. At 2 KHz and below FMDL’s were larger than DLF’s or DLC’s. Above 4 KHz, FMDL’s were smaller than DLF’s or DLC’s. At 2 KHz FMDL’s improved with increasing modulating frequency. The pattern of result suggest that mechanisms play role in frequency discrimination, one based on place of excitation pattern (a place mechanism) and one based on phase locking in the auditory nerve (temporal mechanism). The temporal mechanism only operates below about 4 KHz and within this range it becomes DLF’s and DLC’s. The temporal mechanism is probably sluggish and affects FMDL’s for very low modulation rates .The place mechanism dominates for high frequencies and lower carrier frequencies when stimuli are frequency modulated at high rate.

Frequency Discrimination as a function of frequency and sensation level;

Wier and Green (1976) measured the frequency discrimination for frequencies from 200 to 8000 Hz and for sensation levels from 5 to 80 dB using pulsed sinusoids as stimuli in an adaptive two interval forced choice procedure. The result showed frequency discrimination to be dependent on both frequency and sensation level. The Weber fraction ∆f/f is non monotonic function of frequency between 1000 and 2000 Hz for all sensation levels.

There is orderly dependence of frequency discrimination on sensation levels for pulsed sinusoids. The linear functions are steepest at high sensation levels and decreased as sensation level is decreased. The effect of sensation level is greatest at low frequency and decreased at high frequencies. The result is in agreement with the hypothesis that high frequency information is entirely depended on place of excitation.

Temporal effect in the frequency discrimination:

Frequency discrimination for tones varies with tonal duration. The DLF for signals with duration of more than 150 msec is fairly constant. For less than 150 msec, there is progressive increase in the size of the DLF. (Turball 1944; Chistovichs1960; Cardoz 1962). This effect seems to due to physical characteristics of short tones. That is the band width of the signal increases as its duration decreases. The resulting uncertainty of the signal is manifested as an increase in the size of DLF. Both normal and hearing impaired subjects show this inverse relationship between signal duration and size of DLF.

Geugel (1973) hypothised one cause for high correlation as larger DLF’s in SN loss cases than normal hearing subjects indicates frequency uncertainty in the perception of pure tones. Hence, long duration signals will not sound like pure tones instead they sound rough, buzzing or noisy. The degree of uncertainty varies among hearing impaired subjects depending on their nature of impairment. Reducing the signal duration to 50 msec, and further increases frequency uncertainty

Frequency Discrimination and Speech perception:

Slight correlations have been reported between word recognition and frequency DLs (Di Carlo 1962; Ross et al 1965), consistent with the findings of the study by Tyler et al (1982). In this study it is indicated that hearing impaired listeners had only a small loss of speech understanding in quiet. The result of this study also indicates that the week relationship of speech must certainly depend on numerous underlying properties of frequency, intensity, and temporal coding and it is therefore not surprising that any single factor would not demonstrate high correlation with word identification.

Frequency discrimination in Hearing Impaired Listeners:

It has been shown that frequency resolution (Pick et al 1977; Florentine et al 1980; Tyler et al 1980) and frequency discrimination of constant tone can be altered in listeners with cochlear hearing loss. Only limited data are available on the frequency discrimination of tones changing in frequency in the hearing impaired.

Frequency resolution is the ability to resolve frequency components of stimulus. This could be important in analyzing speech sounds in determining the number and frequency of formants. Frequency discrimination is the ability to discriminate successively presented stimuli that differ in their frequency content. This could be important in determining if the fundamental pitch of one speech sounds is higher or lower compared to some previous sound. The frequency content of speech is dynamic and frequency resolution and discrimination must be performed on formants that are changing in frequency.

Theories of frequency discrimination:

Two mechanisms involved in detecting the DL.

·         The subject may detect shifts in the place of maximum excitation in the cochlear place theory.

·         Temporal information- frequency theory- the subject discriminates the two tones by using some intervals between the neural firings

Place Theory:

Zwicker (1970) has attempted to account for frequency discrimination in terms of changes in the excitation pattern evoked by the stimulus when the frequency altered. Generally regarded as place model, even though the excitation level might be coded partly in the timing of neural impulses. According to him a change in frequency will be detected whenever the excitation level at some point, the pattern changes by more than a certain threshold value. He suggested that this value was about 1 dB. The change in the excitation level is always greatest on the steeply sloping low frequency side of the excitation pattern. This model predicts that the frequency DL at any given frequency should be constant fraction of critical band at that frequency.

FMDL’s do confirm well to the prediction of the model. But DLF’s vary more with frequency than predicted by the model. At low frequencies DLF’s are smaller than predicted, while at high frequency they are slightly larger than predicted (Moore and Glasberg, 1986)

Herning (1966) has pointed out a problem with the measurement of frequency DL’s at high frequencies. The frequency change may be accompanied by correlate loudness changes. These loudness changes may provide observers with usable cues in detection of frequency changes.

In summary, Zwicker’s model predicts that:

1.      Frequency DLs should vary with frequency and random variations in level should markedly increase frequency DLs

2.      DLF vary with frequency than the critical band and the effect of randomizing level is smaller than predicted except at high frequencies.

3.      FMDL’s data are generally consistent with Zwicker’s model.

4.      The results are consistent with the idea that DLF’s are determined by temporal information (phase locking for frequencies up to about 4 to 5 KHz and by place information above that). The precision of phase locking decreases with increasing frequency above 1 to 2 KHz and it is completely absent above about 5 KHz.

Temporal/Frequency Theory:

Rutherford (1886) each cell in cochlea responded to every tone and that frequency information was carried only the frequency of the neural impulses.

DLF- Clinical application:

Differential sensitivity measure can be used in the phenomena of diplacusis binauralis. If we present the tone of constant frequency to one ear and ask him to adjust the frequency of the tone presented to the opposite ear until the two tones sound equal in pitch. If he is a normal listener, he will set the second tone to the frequency of the first plus or minus the associated DL. A listener with diplacusis will set the frequency of the 2^nd tone by more than DL i.e. they tend to hear two widely separated pitches equal. In short diplacusis phenomena is demonstrated in a listener with a hearing loss and seems to confirm a recruiting type of deafness (Hirsh 1952)

Neil and Elliot (1970) demonstrated the effect of DLF on ears with adaptation. There are changes reported in the quality of auditory experience produced by an adapting stimulus which increase with duration. These have an effect on DLF causing shift in pitch. They obtained data under normal and adapted conditions at two frequencies (250 Hz, 1 KHz), two intensities (40 dB SL and 60 dB SL) and with two mode of presentation.

Berndt (1967) have a description of the effect of DLF after exposure to noise. Measures of threshold and DLF were obtained at 1, 2, and 4 KHz before and after exposure to WBN. Stimuli were presented at 10 and 40 dB SL. No differences between pre and post exposure individual were obtained at 40 dB SL or greater at any frequency. However, at low SL’s a differential effect on JND owing to noise exposure was seen. At 2 KHz 10% impairment in the JND existed in the later stage of recovery. At 1 KHz‘s post exposure JND’s were about 40% greater than pre exposure values. It would be interesting to examine the effect of auditory fatigue upon such measures as discrimination. It might provide useful information concerning the fatigue process and their manifestation and quite possibly may also increase our understanding of the mechanism of the intensity frequency discrimination processes as well.

Meweman (1954) conducted a study of monaural frequency discrimination in cases with Menier’s disease. He showed that the groups mean values for DLF in subject with Menier’s disease were substantially greater than values for normal especially at the higher frequencies.

Test Based on DLF:

Cambell (1970) developed a test called Frequency Increment Sensitivity Test (FIST) which is audiometric test using incremental variation in the presentations and scoring method analogous to the SISI test.

A range of frequency increment and sensation levels are used for test. Here, a continues tone was used in which frequency will be changed or in increment of frequency will be employed every 5 sec. this frequency variation was 200 msec in duration. The difference between the maximum frequency during the increment and the base frequency was the measure of frequency size which was represented as the percentage of the base frequency. If the subject gets score less than 40% indicates cochlear pathology. If it is higher either it can be cochlear pathology or normal hearing.

CONCLUSION:

• Ø  There are many differences in studies of intensity and frequency discriminations.
• Ø  The data for intensity discrimination of pulse sinusoids at various frequencies and sensation levels are different than detection of amplitude modulation.
• Ø  Intensity discrimination dependent on SL as well as test frequency. As SL increases, DLI improves; there is near miss to Weber law, DLI is almost constant till 8 KHz, and becomes poor above this frequency.
• Ø   Both normal hearing and cochlear hearing impaired subject’s exhibits near miss to Weber’s Law.
• Ø   Both high frequency and low frequency side of the excitation pattern is necessary for DLI, but high frequency side of the excitation pattern is more important than low frequency.
• Ø  At a given SL, the Weber fraction for frequencies in regions of cochlear loss may be considerably better than normal.
• Ø  The frequency discrimination is dependent on both signal intensity and frequency; changes in signal intensity have a greater effect on at low frequencies than at high frequencies.
• Ø  At low frequency, frequency discrimination is primarily determined by the place mechanism.
• Ø  Frequency resolution and frequency discrimination of constant tones is altered in listeners with cochlear hearing impairment. Frequency discrimination depends primarily on temporal coding and frequency resolution on place coding.
• Ø  Frequency discrimination, particularly by transitions, can be drastically reduced in some hearing impaired listeners. This is not easily predicted on the basis of pure tone thresholds.
• Ø  Studies report slight correlation between SDS and DLF
• In general, intensity and frequency discrimination are dependent on SL and test frequencies. Both aspects are affected in cochlear hearing impaired listeners. The results vary depending on the type and pattern of hearing loss. The DL test of recruitment is based on the measurement of the rate of change in loudness as intensity increases. This rate of change is usually greater in recruiting ears. Clinically these measures can be used to differentiate between cochlear and retro cochlear hearing impaired ears.